
TzTech003 - 校园、充电
【特辑】小周周报第003期,总结放假之前的生活和个人的探索。

【特辑】小周周报第003期,总结放假之前的生活和个人的探索。

小周周报第002期,总结在微信公众号等地方看到的各种感兴趣的文章,内容。

小周周报第001期,总结在微信公众号等地方看到的各种感兴趣的文章,内容。

通过Tampermonkey脚本将浙江大学iZJU PeopleSoft系统的课表导出为ICS格式文件,并导入到各种日历应用的详细教程。

记录高中三年从军训开始到疫情期间的校园生活点滴,包含学习经历、同学友谊、摄影兴趣等青春回忆。
纪念。
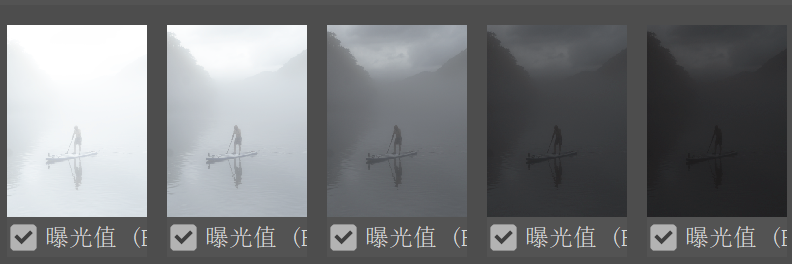
探讨AEB技术在手机摄影中的应用与效果,特别是与手机原生Raw文件的对比分析。

在新的一年里,像鲁迪乌斯一样勇敢面对人生起伏,在困顿时寻找转折点,在顺利时珍惜身边人。面对算力封锁的挑战,我们要开辟新航路。

从艾恩葛朗特开始,到重温EVA,参与多场音乐演出,告别相机拥抱音乐,在高三的路上思考成长与艺术的意义。

2023年,尘埃落定,闲下来后,又想起了某些回忆……